Cauchy and First Sylow Theorem.

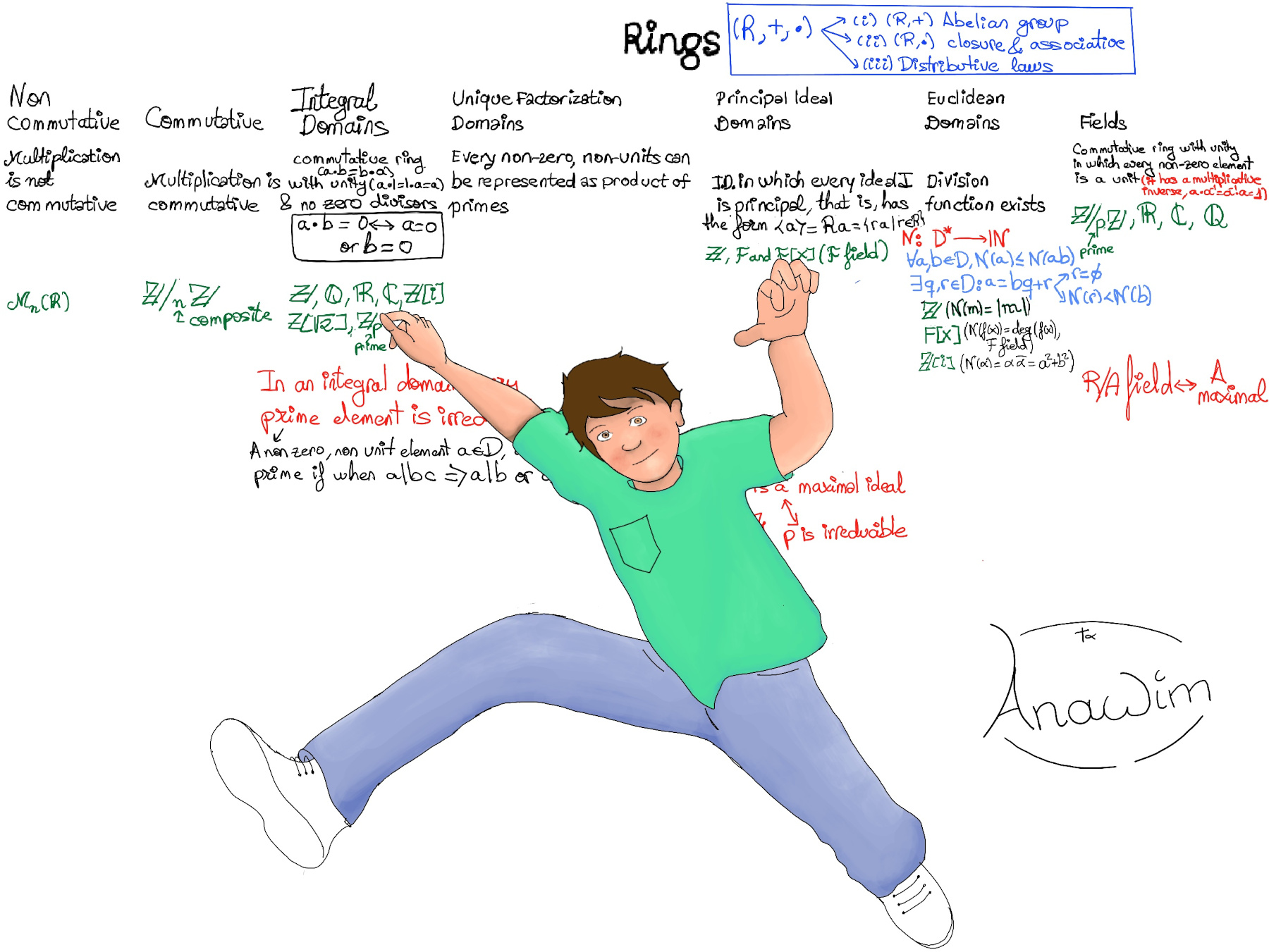
2nd Law. Common sense is not common; lies are abundant and spreading, but the truth is hard to find -Do not kill/cancel the messenger-; nothing good comes easy, it requires sacrifice; and don’t ever underestimate the power of ignorance and stupidity, Apocalypse, Anawim, #justtothepoint.

Recall

Definition. Any two elements a and b of a group G are said conjugate if xax^-1 = b for some x ∈ G.

Conjugacy is an equivalent relation on G, and the conjugacy class of “a”, denoted by cl(a) ={xax^-1 | x ∈ G} is the equivalence class of “a” under conjugacy, and therefore we could partition any group G into disjoint conjugacy classes.

The Class Equation. Let G be a nontrivial finite group. Suppose that a₁, a₂, ···, a_k are the representatives of the conjugacy classes that have size > 1. Then, $|G| = |Z(G)|+\sum_{i=1}^k |G:C(a_k)|$

Sylow’s First Theorem

Sylow’s First Theorem. Let G be a finite group and p be a prime. If p^k | |G| for some integer k ∈ ℤ, then G has a subgroup of order p^k, i.e., ∃H ≤ G such that |H| = p^k.

Proof.

Let’s use induction on |G|.

If |G| = 1 ⇒ G is the trivial group, G = {e} ⇒ p^k ɫ |G| = 1 for any integer k, so the theorem is obviously true.

Let’s assume that the theorem holds for all groups of order less than |G|.

Let’s assume p^k | |G| for some integer k ∈ ℤ, then if there are some proper subgroup of G, say H < G, |H| = p^k (H is itself a group of order less than |G|), then, by our inductive hypothesis, H has a subgroup of order p^k, namely H itself, and the statement holds.

Let’s assume that there is no proper subgroup H of G such that p^k ɫ |H|. By the class equation, $|G| = |Z(G)|+\sum_{i=1}^k |G:C(a_k)|$ where this sum is done over representatives of conjugacy classes a_k such that a_k ∉ Z(G).

Since p^k | |G| = |G:C(a)|·|C(a)|, and p^k does not divide |C(a)| (By assumption, there is no such subgroup and C(a) is a subgroup of G) ⇒ p | |G:C(a)| ∀a ∉ Z(G) ⇒ [Cauchy's Theorem for Abelian Group. Let G be a finite Abelian group and let p be a prime that divides |G| ⇒ G has an element of order p] Z(G) is a finite Abelian group, p | |Z(G)|🚀 ⇒ ∃z ∈ Z(G) of order p.

🚀It follows from the class equation, $|G| = |Z(G)|+\sum_{i=1}^k |G:C(a_k)|$ and p | |G:C(a)| ∀a ∉ Z(G), p^k | |G| ⇒ p | |G| - $\sum_{i=1}^k |G:C(a_k)|$ = |Z(G)|

Let Z = ⟨z⟩ be the cyclic group generated by z ⇒ [Because any subgroup of Z(G) is normal. Remember that a group is normal if it is closed under conjugation] Z = ⟨z⟩ is a normal subgroup of G and we may form the factor or quotient group H = G/Z = G/⟨z⟩ ⇒ Since the order of H is given by |H| = |G|/|Z| = |G|/p ⇒ |H| < |G| ⇒ [The induction hypothesis applies, |H| < |G|, p^k | |G| and p^k-1| |H| = |G|/|Z| = |G|/p] there exists a subgroup of H = G/Z = G/⟨z⟩ of order p^k-1 ⇒ [Correspondence Theorem, Let G be a group, N ⊲ G be a normal subgroup, then every subgroup of the quotient group G/N is of the form S/N = {sN: s ∈ S}, where N ≤ S ≤ G] this subgroup has the form S/⟨z⟩ where S is a subgroup of G ⇒ |S/⟨z⟩| = p^k-1 and |⟨z⟩| = p ⇒ |S| = p^k∎

Example

• Let G be a finite group, |G| = 2³·3²·5³·7 ⇒ [Sylow’s First theorem] G must have at least one subgroup of each of the following orders: 2 (2¹), 4 (2²), 8 (2³), 3 (3¹), 9 (3²), 5 (5¹), 25 (5²), 125 (5³), and 7 (7¹).

Definition. Let G be a finite group, and let p be a prime dividing the order of G. Then, a Sylow p-subgroup of G is a maximal p-subgroup of G, that is, p^k divides |G| and p^k+1 does not divide |G|. Let Syl_p(G) denote the set of Sylow p-subgroups of G.

Examples

• Let G = ℤ₁₂, ℤ₁₂ = 2²·3 then ⟨4⟩ ≋ ℤ₃ is the unique Sylow 3-subgroup of ℤ₁₂ and ⟨3⟩ ≋ ℤ₃ is the unique Sylow 2-subgroup of ℤ₁₂.

• In our previous example, any subgroup of order 8 is a Sylow 2-subgroup of G, any subgroup of order 125 is a Sylow 5-subgroup of G and any subgroup of order 7 is a Sylow 7-subgroup of G; in other words, p^k divides |G|, but p^k+1 does not divide |G|, i.e., the largest power of p consistent with Lagrange's theorem.

• Let G = S₃, with |S₃| = 6 = 2·3. Then, S₃ has a unique Sylow 3-subgroup, namely A₃, but three distinct Sylow 2-subgroups: ⟨12⟩, ⟨13⟩, and ⟨23⟩.

• Let G = A₄, with |A₄| = 12 = 2²·3. Then, A₄ has a unique Sylow 4-subgroup, namely the Klein 4-group V₄ = {1, (12)(34), (13)(24), (14)(23)}, and four Sylow (cyclic) 3-subgroups of order 3.

Cauchy’s Theorem. Let G be a finite group and let p be a prime that divides the order of G. Then, G has an element of order p.

Proof.

Let G be a finite group and let p be a prime that divides the order of G, p | |G| ⇒ [Sylow’s First Theorem. Let G be a finite group and p be a prime. If p^k | |G| for some integer k ∈ ℤ, then G has a subgroup of order p^k, i.e., ∃H ≤ G such that |H| = p^k.] G has a subgroup of order p = p^k for k = 1 ⇒ [Since any subgroup of order p prime is cyclic] G has an element of order p.

p needs to be prime. Klein 4-group does not contain an element of order 4 (4 | 4), but it contains an element of order 2.

Bibliography

1. NPTEL-NOC IITM, Introduction to Galois Theory.
2. Algebra, Second Edition, by Michael Artin.
3. LibreTexts, Abstract and Geometric Algebra, Abstract Algebra: Theory and Applications (Judson).
4. Field and Galois Theory, by Patrick Morandi. Springer.
5. Michael Penn (Abstract Algebra), and MathMajor.
6. Contemporary Abstract Algebra, Joseph, A. Gallian.
7. Andrew Misseldine: College Algebra and Abstract Algebra.