Properties of integrals

Never underestimate the power of stupid people in large groups, George Carlin.

All those who seem stupid are stupid, and so are half of those who don’t seem so, Quevedo.

Recall

Antiderivatives are fundamental concepts in calculus. They are the inverse operation of derivatives.

Given a function f(x), an antiderivative, also known as indefinite integral, F is the function that can be differentiated to obtain the original function, that is, F’ = f, e.g., 3x² -1 is the antiderivative of x³ -x +7. Symbolically, we write F(x) = $\int f(x)dx$.

The process of finding antiderivatives is called integration.

The Fundamental Theorem of Calculus states roughly that the integral of a function f over an interval is equal to the change of any antiderivate F (F'(x) = f(x)) between the ends of the interval, i.e., $\int_{a}^{b} f(x)dx = F(b)-F(a)=F(x) \bigg|_{a}^{b}$

Properties of integrals

1. The integration of the sum of two functions is equal to the sum of the integration of the individual functions, $\int (f(x) + g(x))dx = \int f(x)dx + \int g(x)dx$, e.g., $\int (8x -5)dx = \int 8x dx -\int 5 dx = \frac{8x^2}{2} + c_1 -5x + c_2 = 4x^2-5x+c$.

2. $\int cf(x)dx = c\int f(x)dx$ where c is a constant, e.g., $\int 7·cos(x)dx = 7\int cos(x)dx = 7(sin(x) + c_1) = 7sin(x) + c_2.$

3. Linearity of Integrals, $\int (a·f(x) + b·g(x))dx = a·\int f(x)dx + b·\int g(x)dx$. In other words, you can split the integral of a sum into the sum of integrals and pull constants out of the integral, e.g., $\int (e^{3x}-x^7)dx = \int e^{3x}dx -\int x^7dx = \frac{e^{3x}}{3}-\frac{x^8}{8}+c.$

4. When the limits of integration are the same, the integral value is equal to 0, regardless of the integrand function, $\int_{a}^{a} f(x)dx = 0$. In essence, it’s the integral over a single point.

5. Integrating Definite Integral Backwards. $\int_{a}^{b} f(x)dx = -\int_{b}^{a} f(x)dx$, and this rule is very important, $\int_{a}^{b} f(x)dx = F(b)-F(a) = -(F(a) - F(b)) = -\int_{b}^{a} f(x)dx$, that is, our Fundamental Theorem of Calculus always applies and this property always work, i.e., it is not necessary that a < b < c.

6. Definite Integrals on Adjacent Intervals. Let a < b < c, $\int_{a}^{b} f(x)dx + \int_{b}^{c} f(x)dx = \int_{a}^{c} f(x)dx$. In words, we can break up a integral into pieces or smaller intervals with the same integrand, e.g., $\int_{4}^{7} sin(3x)dx + \int_{-2}^{4} sin(3x)dx = \int_{-2}^{7} sin(3x)dx = -\frac{1}{3}(cos(3x))\bigg|_{-2}^{7} = \frac{1}{3}(-cos(21)-(-cos(-6))) = \frac{1}{3}(-cos(21)-(-cos(6))) = \frac{1}{3}(cos(6)-cos(21))≈0.50263$. 👁Recall that cos(θ) = cos(-θ).

7. Symmetry. If f is an even function, then $\int_{-a}^{a} f(x)dx = 2\int_{0}^{a} f(x)dx.$ If f is an odd function, then $\int_{-a}^{a} f(x)dx =0.$

   Suppose f is an even function, $\int_{-a}^{a} f(x)dx$ =[Definite Integrals on Adjacent Intervals] $\int_{-a}^{0} f(x)dx + \int_{0}^{a} f(x)dx$= [Integrating Definite Integral Backwards] $-\int_{0}^{-a} f(x)dx + \int_{0}^{a} f(x)dx$ =[Change of variables u = -x, du = -dx, u₁ = 0, u₂ = a] $\int_{0}^{a} f(-u)du + \int_{0}^{a} f(x)dx$ =[f is an even function, f(-x) = f(x)] $\int_{0}^{a} f(u)du + \int_{0}^{a} f(x)dx = 2\int_{0}^{a} f(x)dx$

   Suppose f is an odd function, $\int_{-a}^{a} f(x)dx$ =[Definite Integrals on Adjacent Intervals] $\int_{-a}^{0} f(x)dx + \int_{0}^{a} f(x)dx$=[Integrating Definite Integral Backwards] $-\int_{0}^{-a} f(x)dx + \int_{0}^{a} f(x)dx$ =[Change of variables u = -x, du = -dx, u₁ = 0, u₂ = a] $\int_{0}^{a} f(-u)du + \int_{0}^{a} f(x)dx$= [f is an odd function, f(-x) = -f(x)] $-\int_{0}^{a} f(u)du + \int_{0}^{a} f(x)dx = 0.$

8. Comparison Theorem. If f(x) ≥ 0, ∀x ∈ [a, b] ⇒ $\int_{a}^{b} f(x)dx ≥ 0.$ If f(x) ≤ g(x), ∀x ∈ [a, b] then $\int_{a}^{b} f(x)dx ≤ \int_{a}^{b} g(x)dx$ where a < b. In a physic interpretation, if I am travelling more slowly than you, then you will reach further than I do. If m and M are constant such that m ≤ f(x) ≤ M, ∀x ∈ [a, b] ⇒ m(b-a) ≤ $\int_{a}^{b} f(x)d(x)$ ≤ M(b -a)

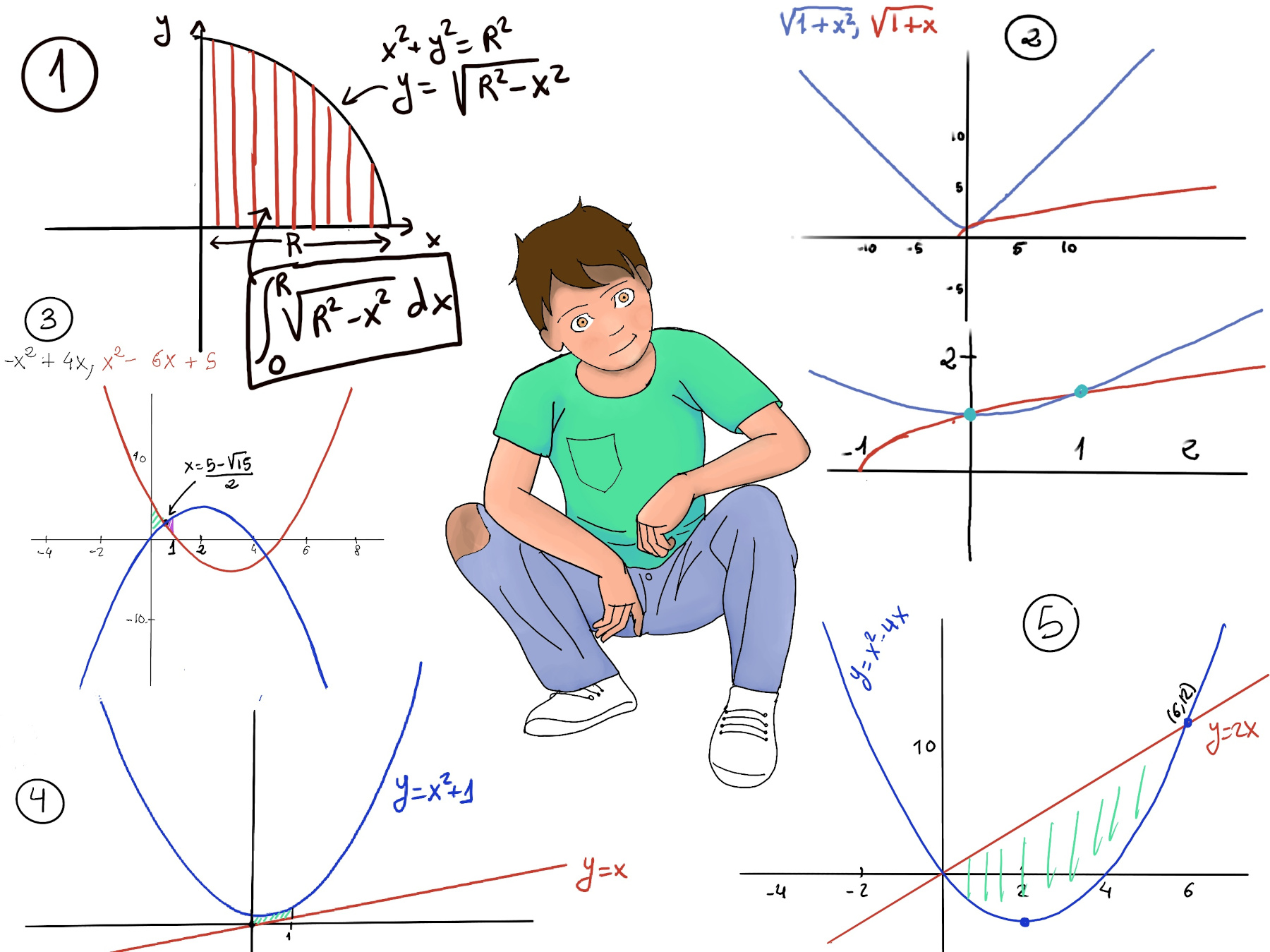
   Example: f(x) = $\sqrt{1+x^2}$ and g(x) = $\sqrt{1+x}$ over the interval [0, 1]. Initially, when graphed on any plotting software, f(x) appears to be above g(x). We need to zoom in to see that g(x) is above f(x) on the interval [0, 1] (Figure 2) ⇒ $\int_{0}^{1} g(x)dx ≥ \int_{0}^{1} f(x)dx$

    

   Example: x ≥ 0 ⇒[The exponential function is strictly increasing] e^x ≥ 1 ⇒ $\int_{0}^{b} e^xdx ≥ \int_{0}^{b} 1dx$. $\int_{0}^{b} e^xdx = e^x\bigg|_{0}^{b}=e^b - 1$ 🚀.

   Futhermore, $\int_{0}^{b} 1dx = b ⇒ e^b - 1 ≥ b ↭ e^b ≥ 1 + b$ where b ≥ 0.

   Analogously, taking into consideration that e^x ≥ 1 + x, x ≥ 0, then $\int_{0}^{b} e^xdx =🚀 e^b -1 ≥ \int_{0}^{b} (1 + x)dx = (x + \frac{x^2}{2})\bigg|_{0}^{b} = b + \frac{b^2}{2}$. In conclusion, e^b -1 ≥ $b + \frac{b^2}{2} ↭ e^b ≥ 1 + b + \frac{b^2}{2}$ where b ≥ 0.

9. Integration by substitution or change of variables is a technique used to simplify integrals by introducing a new variable. It is particularly useful when the integrand contains a complicated expression or when the substitution helps to reveal an easier form to calculate the integral, $\int_{u_1}^{u_2} g(u)du = \int_{x_1}^{x_2} g(u(x))u’(x)dx$ where u = u(x), du = u’(x)dx, u₁ = u(x₁), and u₂ = u(x₂). This property only works if u’ does not change signs

Examples

• $\int_{0}^{2} 2xe^{x^2}dx$ =[1. Choose a suitable substitution, e.g. u = x² such that it simplifies the integral. 2. Find the differential of u with respect to x, e.g. du = 2xdx, u₁ = 0, u₂ = 4]. 3. Rewrite and evaluate the integral in terms of the new variable, = $\int_{0}^{4} e^{u}du = e^u\bigg|_{0}^{4} = e^4 -e^0 = e^4 -1 ≈ 53.598.$

• $\int_{0}^{1} x\sqrt{1-x^2}dx$ =[u = 1-x², du = -2xdx, u₀ = 1, u₁ = 0] = $\frac{-1}{2}\int_{0}^{1} \sqrt{u}du = \frac{-1}{2}\frac{u^{\frac{3}{2}}}{\frac{3}{2}} = \frac{-1}{3}u^{\frac{3}{2}}\bigg|_{1}^{0} = 0-(-\frac{1}{3}) = \frac{1}{3}.$

• $\int_{1}^{2} (x^3 + 2)^5x^2dx =$[u = x³+2, du = 3x²dx, u₁ = 1³ + 2 = 3, u₂ = 2³ + 2 = 10] $\int_{3}^{10} u^5\frac{1}{3}du = \frac{1}{18}u^6\bigg|_{3}^{10} = \frac{1}{18}(10^6-3^6) = 55515.0555556.$

• $\int \frac{1}{1+\sqrt{x}}dx =$[$u = \sqrt{x}, \frac{du}{dx} = \frac{1}{2\sqrt{x}} ⇒ dx = du·2\sqrt{x} = 2udu$] = $\int \frac{2udu}{1+u} = 2\int \frac{udu}{1+u} = 2(\int \frac{u+1-1}{1+u}du) = 2(\int \frac{u+1}{1+u}du + \int \frac{-1}{1+u}du) = 2(\int du - \int \frac{-1}{1+u}du) = 2u -2ln|1+u| + C = 2\sqrt{x}-2ln|1+\sqrt{x}| + c.$

• Calculate the area of a circle (Figure 1). $Area = 4·\int_{0}^{R} \sqrt{R^2-x^2}dx =$[x = Rcos(θ), dx = -Rsin(θ)dθ, 0 = cos(θ₁) ⇒ θ₁ = ^π⁄₂, R = Rcos(θ₂) ⇒ cos(θ₂) = 1 ⇒ θ₂ = 0] $4·\int_{\frac{π}{2}}^{0} \sqrt{R^2-R^2cos^2(θ)}(-Rsin(θ)dθ) = 4·\int_{\frac{π}{2}}^{0} R\sqrt{1-cos^2(θ)}(-Rsin(θ)dθ) = 4·\int_{\frac{π}{2}}^{0} R\sqrt{sin^2(θ)}(-Rsin(θ)dθ) = 4·\int_{\frac{π}{2}}^{0} Rsin(θ)(-Rsin(θ)dθ) = 4R^2\int_{0}^{\frac{π}{2}} sin^2(θ)dθ = 4R^2\int_{0}^{\frac{π}{2}} \frac{1-cos(2θ)}{2}dθ = 4R^2\int_{0}^{\frac{π}{2}} \frac{1}{2}dθ -4R^2\int_{0}^{\frac{π}{2}} \frac{-cos(2θ)}{2}dθ = 4R^2·\frac{1}{2}·θ -4R^2·\frac{1}{2}\frac{sin(2θ)}{2} = 2R^2θ-R^2sin(2θ)\bigg|_{0}^{\frac{π}{2}} = 2R^2\frac{π}{2}-R^2sin(π)+R^2sin(0)= R^2π$

• 💀$\int_{-1}^{1} x^2dx =$[u = x², du = 2xdx ⇒ dx = du/2x, u₁ = (-1)² = 1, u₂ = 1] $\int_{1}^{1} u\frac{1}{2\sqrt{u}}du = \frac{1}{2}\frac{u^{\frac{3}{2}}}{\frac{3}{2}} =\frac{u^{\frac{3}{2}}}{3}\bigg|_{-1}^{1} = 0$ ⊥ x² is a positive parabola and its area is greater than zero. The problem is that u' = 2x does change signs because 2x > 0 if x > 0, 2x < 0 if x < 0.

$\int_{-1}^{1} x^2dx =\frac{x^3}{3}\bigg|_{-1}^{1} = \frac{1}{3}-\frac{-1}{3}=\frac{2}{3} ≈ 0.66667.$

Bibliography

1. NPTEL-NOC IITM, Introduction to Galois Theory.
2. Algebra, Second Edition, by Michael Artin.
3. LibreTexts, Calculus. Abstract and Geometric Algebra, Abstract Algebra: Theory and Applications (Judson).
4. Field and Galois Theory, by Patrick Morandi. Springer.
5. Michael Penn, and MathMajor.
6. Contemporary Abstract Algebra, Joseph, A. Gallian.
7. YouTube’s Andrew Misseldine: Calculus. College Algebra and Abstract Algebra.
8. MIT OpenCourseWare 18.01 Single Variable Calculus, Fall 2007 and 18.02 Multivariable Calculus, Fall 2007.
9. Calculus Early Transcendentals: Differential & Multi-Variable Calculus for Social Sciences.