Exponential functions

“Don’t patronize me. My face makes onions and blind kids cry, vultures vomit, grannies ran past me, ghosts shiver, Medusa turn to stone, and the mother of all assholes very proud,” Apocalypse, Anawim, #justtothepoint.

The reports of my death have been greatly exaggerated, Mark Twain.

Recall

Definition. A function f is a rule, relationship, or correspondence that assigns to each element of one set (x ∈ D), called the domain, exactly one element of a second set, called the range (y ∈ E).

The pair (x, y) is denoted as y = f(x). Typically, the sets D and E will be both the set of real numbers, ℝ.

Graphing functions involves the visual representation of a curve that reflects the behavior of a mathematical function on a coordinate plane, also known as the Cartesian plane. It is a two-dimensional space defined by two axes - the x-axis (horizontal) and the y-axis (vertical).

A systematic approach to graphing a function begins by constructing a table containing selected input values and their corresponding output values. By extending this table, we can plot the points on the coordinate plane and observe the pattern they form. Connecting these points reveals the shape of the curve, allowing us to gain insights into the behavior of the function across its domain. This process provides a visual means of understanding the relationships between different variables and aids in interpreting the overall characteristics of the function.

Exponential functions

Apparently the ruler of India many years ago was extremely delighted with the newly created game of chess and he generously offered the inventor any reward of his choosing.

The wise man appeared humble when he asked for a single grain of rice to be placed on the first square of the chessboard on the first day, two grains on the second square on the next day, four grains on the third, and so on, doubling the amount each time until all 64 squares had been filled.

The king, perplexed by what appeared to be a trivial request for such a captivating game, swiftly agreed and instructed his treasurer to fulfill the agreement. However, a week later, the inventor returned to the king, inquiring about his yet-to-be-received reward. The king, infuriated by the apparent disobedience of the treasurer, promptly summoned him for an explanation.

The treasurer clarified that the stipulated sum could not be paid, as the quantity of grains required had surpassed the entire kingdom’s grain supply. The astounding reality is that the 64th square requires 9,223,372,036,854,780,000 grains of rice. That’s 9.223 quintillion! There isn’t enough rice in the world for this one square alone, never mind the rest of the board.

Upon absorbing this information, the king pondered the situation and eventually made a decision. In what some might consider a ruthless act, he chose to have the inventor executed. The intention behind this harsh decision was to serve as a stark lesson on the consequences of attempting to outsmart the king.

The exponential function is a type of mathematical function which is helpful in finding the growth or decay of a population, modelling the lifetime of a certain product, exponential growth of cancer cells or bacteria, etc. For instance, a population of rabbits doubles every month, we would have 2, then 4, then 8, 16, 32, 64, 128, 256, etc! Exponential growth generally starts relatively slow but, once it gets going, it grows very, very quickly.

An exponential function is a mathematical function of the form f(x) = b·a^x, where the independent variable is the exponent, and a and b are constants. a is called the base of the function and it should be a positive real number (a>0).

Examples are 2^x, 7^x, and (¹⁄₄)^x. There are three kinds of exponential functions depending on whether a > 1, a = 1, or 0 < a < 1 -Figure 1.a.- The more important example is the natural exponential function, f(x) = e^x where e is the Eulers Number, a mathematical constant approximately equal to 2.71828.

1. If a > 1, it can be observed that as the exponent increases, the curve get steeper. As x increases, f(x) heads to infinity, $\lim_{x \to ∞} a^x = ∞$. As x decreases, f(x) heads to zero, $\lim_{x \to -∞} a^x = 0$. It is strictly increasing ((e^x)’ = e^x > 0, (a^x)’ = a^x·ln(a) > 0) and has a horizontal asymptote along the x-axis.

   It is important to realize that as x approaches negative infinity, the results become very small but never actually attain zero, e.g., 2^-5 ≈ 0.03125, 2^-15 ≈ 0.00003052. Besides, the base of an exponential function determines the rate of growth or decay. For a > 1, the larger the base, the faster the function grows (Figure iii).

    
2. 0 < a < 1. As x increases, f(x) heads to zero, $\lim_{x \to ∞} a^x = 0$. As x decreases, f(x) heads to ∞, $\lim_{x \to -∞} a^x = ∞$. It is strictly decreasing and has a horizontal asymptote along the x-axis.
3. When a = 1, the graph is a horizontal line, y = 1.  

Properties

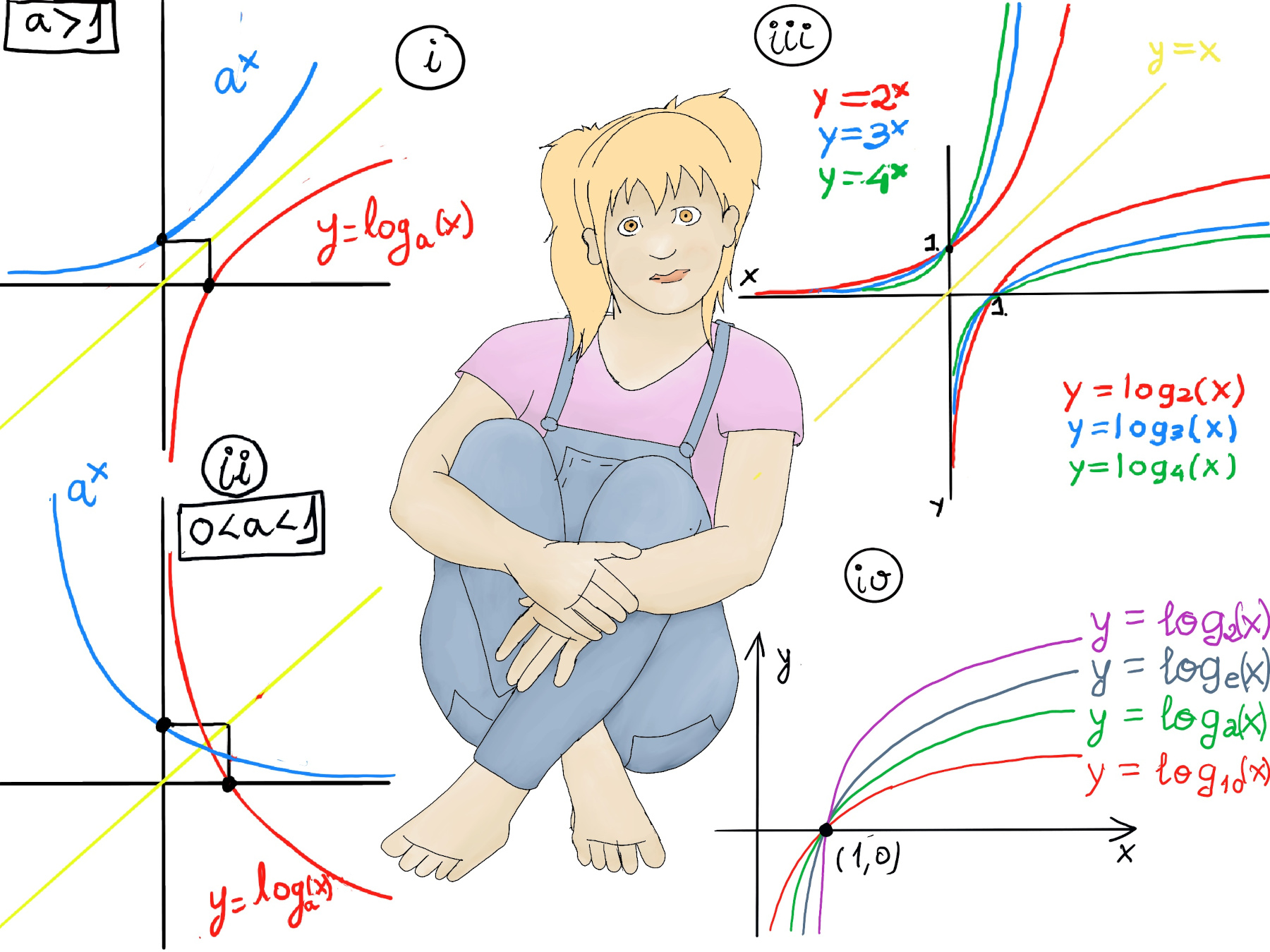
0. It always intersects the y-axis at y=1 (a⁰ = 1), that is, it passes through (0,1). a¹ = a, in other words it passes through (1, a).

1. The domain of an exponential function is the set of all real numbers.

2. It is always positive, a^x > 0, ∀x ∈ ℝ, i.e., the range is the set of all the positive real numbers.

3. If a > 1, the function represents exponential growth. They have graphs that rise rapidly, e.g., 2^x, 2·3^x. If 0 < a < 1, the function represents exponential decay, e.g., (¹⁄₂)^x, 7·(0.9)^x. They have graphs that decrease rapidly.

   Exponential growth is bigger and faster than polynomial growth. This means that, no matter what the degree is on a given polynomial, a given exponential function will eventually be bigger than the polynomial. Formally, for a > 1 and any arbitrary k > 0, $\lim_{x \to ∞} \frac{x^k}{a^x} = 0$ (just apply l’hospital rule several times in succession).

4. Exponent rules. If a, b > 0 and x, y are real numbers, then: a⁰ = 1, a^xa^y=a^x+y, $\frac{a^{x}}{a^{y}}=a^{x-y}$, $(a^{x})^{y}=a^{xy}=(a^{y})^{x}$, $a^{x}b^{x}=(ab)^{x}, (\frac{a}{b})^x=\frac{a^x}{b^x}$.

Exponential Function derivate and series

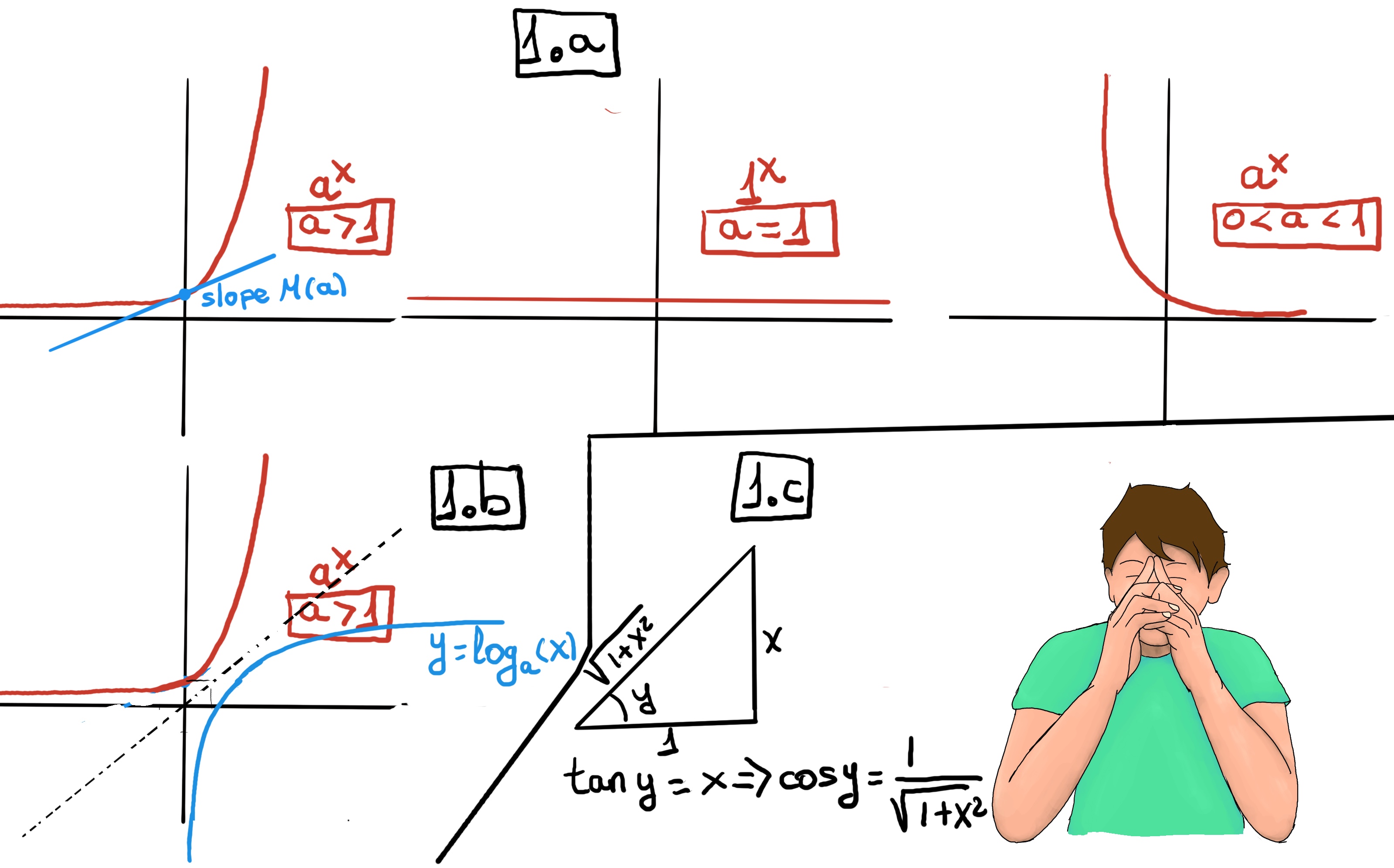
$\frac{d(a^x)}{dx} = \lim_{h \to 0} \frac{a^{x+h}-a^h}{h} = \lim_{h \to 0} \frac{a^xa^h-a^x}{h} = \lim_{h \to 0} \frac{a^x(a^h-1)}{h} = a^x\lim_{h \to 0} \frac{a^h-1}{h} = f’(0)·a^x$. This sucks!😞 We need to know the derivate in order to get the derivate!

Recall that e is the unique positive number for which $f’(0) = \lim_{h \to 0} \frac{e^h-1}{h} = 1$ ⇒ If f(x) = e^x ⇒ f’(x) = e^x·1 = e^x.

f(x) = a^x⇒ [a = e^ln(a)] f(x) = $(e^{lna^{x}}) = e^{(ln(a)·x)}$ ⇒[Chain Rule] f’(x) = $(e^{lna^{x}})·ln(a) = a^x·ln(a),$ hence f'(x) = $a^x·ln(a)$.

• f’(x) = $a^x·ln(a)$ and a^x > 0. If a > 1 ⇒ ln(a) > 0 ⇒ f’(x) > 0 ⇒ f is strictly increasing. If 0 < a < 1 ⇒ ln(a) < 0 ⇒ f’(x) < 0 ⇒ f is strictly decreasing.

• The derivative of the exponential function is the function itself, $(e^{x})'=e^x$ ⇒ e^x > 0, The exponential is strictly increasing.

• $e^x =$[Taylor Series] $\sum_{n=0}^\infty \frac{(x-a)^n}{n!}f^{n}(a)$ =[a = 0, $(e^{x})’=e^x$, e⁰ = 1] $\sum_{n=0}^\infty \frac{x^n}{n!}$. In particular, e = $\sum_{n=0}^\infty \frac{1}{n!}$

Exercises

• Simplify the expression $\frac{27^{2x-3}}{9^{x-4}}$.

$\frac{27^{2x-3}}{9^{x-4}} = \frac{3^{3^{2x-3}}}{3^{2^{x-4}}}$ ⇒[$(a^{x})^{y}=a^{xy}=(a^{y})^{x}$] $\frac{3^{3(2x-3)}}{3^{2(x-4)}} = \frac{3^{6x-9}}{3^{2x-8}}$ ↭[$\frac{a^{x}}{a^{y}}=a^{x-y}$] = $3^{6x-9-(2x-8)} = 3^{4x-1}$

• Solve the equation, (1/4)^x = 64.

(1/4)^x = 64 ↭[$(\frac{a}{b})^x=\frac{a^x}{b^x}$] $\frac{1^x}{4^x} = 64↭ 4^{-x} = 4^3$ ⇒[a = 4 > 1, the exponential is strictly increasing (⇒ one-to-one)] x = -3.

• Solve the equation, 32^(x/3) = 8^(x-12).

$32^{\frac{x}{3}} = 8^{x-12} ↭ 2^{5^{(\frac{x}{3})}} = 2^{3^{(x-12)}}$ ⇒[$(a^{x})^{y}=a^{xy}=(a^{y})^{x}$] $2^{\frac{5x}{3}}=2^{3(x-12)}$ ⇒[a = 2 > 1, the exponential is strictly increasing (⇒ one-to-one)] $\frac{5x}{3} = 3(x-12)↭ 5x = 9(x-12) ↭ 5x = 9x -108 ↭ 4x = 108 ↭ x = \frac{104}{4} = 27.$

• Solve the equation, 3^2x -6·3^x -27 = 0.

Notice that $3^{2x} = 3^{x^{2}}$ ⇒ the given equation is a quadratic equation, let y = 3^x, y² -6y -27 = (y +3)(y -9) = 0 ⇒ There are two options y = -3 or y = 9 ⇒ $3^x = -3⊥, 3^x = 9$ ⇒ x = 2.

Bibliography

1. NPTEL-NOC IITM, Introduction to Galois Theory.
2. Algebra, Second Edition, by Michael Artin.
3. LibreTexts, Calculus. Abstract and Geometric Algebra, Abstract Algebra: Theory and Applications (Judson).
4. Field and Galois Theory, by Patrick Morandi. Springer.
5. Michael Penn, and MathMajor.
6. Contemporary Abstract Algebra, Joseph, A. Gallian.
7. YouTube’s Andrew Misseldine: Calculus. College Algebra and Abstract Algebra.
8. MIT OpenCourseWare 18.01 Single Variable Calculus, Fall 2007 and 18.02 Multivariable Calculus, Fall 2007.
9. Calculus Early Transcendentals: Differential & Multi-Variable Calculus for Social Sciences.