Average Value Theorem

Not everything that can be counted counts and not everything that counts can be counted, Albert Einstein.

Quantity has a quality all its own, Joseph Stalin.

Recall

Antiderivatives are fundamental concepts in calculus. They are the inverse operation of derivatives.

Given a function f(x), an antiderivative, also known as indefinite integral, F, is the function that can be differentiated to obtain the original function, that is, F’ = f, e.g., 3x² -1 is the antiderivative of x³ -x +7 because $\frac{d}{dx} (x^3-x+7) = 3x^2 -1$. Symbolically, we write F(x) = $\int f(x)dx$.

The process of finding antiderivatives is called integration.

The Fundamental Theorem of Calculus states roughly that the integral of a function f over an interval is equal to the change of any antiderivate F (F'(x) = f(x)) between the ends of the interval, i.e., $\int_{a}^{b} f(x)dx = F(b)-F(a)=F(x) \bigg|_{a}^{b}$

Average Function Theorem

One of the most valuable applications of the definite integral is that it provides a way to calculate the average value of a function.

Definition. The average value of a continuous function f(x) over the interval [a, b] is AVG = $\frac{y_1+y_2+···+y_n}{n}$ where y₁, y₂, ···, y_n represent the function values at specific points within the interval [a, b] and n is the total number of points where the function is evaluated within the interval [a, b].

Since integrals are essentially a sum of all of the possible f(x) values, AVG = $\frac{1}{b-a}\int_{a}^{b} f(x)dx$.

Said in a more mathematical way, $\lim_{n \to ∞}\frac{y_1+y_2+···+y_n}{n}$ = $\frac{1}{b-a}\int_{a}^{b} f(x)dx$ where $\int_{a}^{b} f(x)dx$ represents the signed area under the curve of f(x) between x = a and x = b, $\frac{1}{b-a}$ is a scaling factor. It normalizes the integral by dividing it by the length of the interval [a, b], and by doing so, it ensures that the average value is not affected at all by the length of the interval.

Given a function f(x) over the interval [a, b] and a partition P of [a, b] into n sub-intervals, say a = x₀ < x₁ < x₂ < ··· < x_n = b, y₀ = f(x₀), y₁ = f(x₁),···, y_n = f(x_n). The Right Riemann sum approximates the definite integral of a function over a specified interval by using a sum of values from the function evaluated at selected points within that interval, $\sum_{i=1}^n f(x_i)\Delta x$ = (y₁ + y₂ + ··· + y_n)$\Delta x$ →_{[$\Delta x → 0$]} $\int_{a}^{b} f(x)dx$.

Next, let’s divide by b -a, $\frac{(y_1 + y_2 + ··· + y_n)\Delta x}{b-a} → \frac{\int_{a}^{b} f(x)dx}{b-a}$ where $\Delta x = \frac{b-a}{n} ⇒ \frac{\Delta x}{b-a} = \frac{1}{n}$, i.e., $\frac{y_1 + y_2 + ··· + y_n}{n} → \frac{\int_{a}^{b} f(x)dx}{b-a}$.

Average Value Theorem. If f is a continuous function on [a, b], then its average or mean value on [a, b] is given by f_avg = $\frac{\int_{a}^{b} f(x)dx}{b-a}$

Examples

• Let f be the constant function, f(x) = c.

f_avg = $\frac{\int_{a}^{b} f(x)dx}{b-a} = \frac{\int_{a}^{b} cdx}{b-a} = c$, and this is completely expected for an average value.

• Let f(x) = x² over the interval [0, 2]

f_avg = $\frac{\int_{a}^{b} f(x)dx}{b-a} = \frac{\int_{0}^{2} x^2dx}{2-0} = \frac{x^3}{6}\bigg|_{0}^{2} = \frac{2^3}{6}=\frac{4}{3}$

• Let f(x) = 2x -4 over the interval [1, 5]

f_avg = $\frac{\int_{a}^{b} f(x)dx}{b-a} = \frac{\int_{1}^{5} (2x-4)dx}{5-1} = \frac{1}{4}(\frac{2x^2}{2}-4x) = \frac{1}{4}(x^2-4x)\bigg|_{1}^{5} = \frac{1}{4}(25-20-(1-4)) = \frac{8}{4} = 2$

• Let f(x) = $\sqrt{x}$ over the interval [1, 4]

f_avg = $\frac{\int_{a}^{b} f(x)dx}{b-a} = \frac{1}{3}\int_{1}^{4} \sqrt{x}dx = \frac{1}{3}\left[ \frac{2}{3}x^{\frac{3}{2}} \right]_{1}^{4} = \frac{2}{9}(4^{\frac{3}{2}} - 1^{\frac{3}{2}}) = \frac{2}{9}(8 - 1) = \frac{2}{9}(7) = \frac{14}{9}$

• Let f(x) = sin(x) over the interval [0, π]

f_avg = $\frac{\int_{a}^{b} f(x)dx}{b-a} = \frac{\int_{0}^{π} sin(x)dx}{π-0} = \frac{-cos(x)}{π}\bigg|_{0}^{π} = \frac{-cos(π)}{π}+\frac{cos(0)}{π} = \frac{2}{π}$

Find the Average Value with the Mean Value Theorem for Integrals

The Mean Value Theorem for Integrals. If f is a function that is continuous on [a,b] and differentiable on (a,b), then there is at least one point c ∈ [a, b] such that $f(c) = \frac{1}{b-a}\int_{a}^{b} f(x)d(x)$ ⇒ $\int_{a}^{b} f(x)d(x) = f(c)(b-a)$

This is very similar to our previous statement, f_avg = $\frac{\int_{a}^{b} f(x)dx}{b-a}⇒\int_{a}^{b} f(x)dx = f_{avg}(b-a) = f(c)(b-a)$. The Mean Value Theorem for Integrals is telling us that there is a number c∈ [a, b] such that f_avg = f(c), that is, if f(x) is a continuous function then somewhere in [a, b] the function will take on its average value.

Solved exercises

• Average height of a point on a unit semi circle with respect to the x axis.

$\frac{1}{b-a}\int_{a}^{b} f(x)dx = \frac{1}{1-(-1)}\int_{-1}^{1} \sqrt{1-x^2}dx$ =[$\int_{-1}^{1} \sqrt{1-x^2}dx = \frac{π}{2}$ because it is the area of a semicircle 🚀, that is, half the area of a circle] $\frac{1}{2}(\frac{π}{2}) = \frac{π}{4}$ (Figure 1.a.).

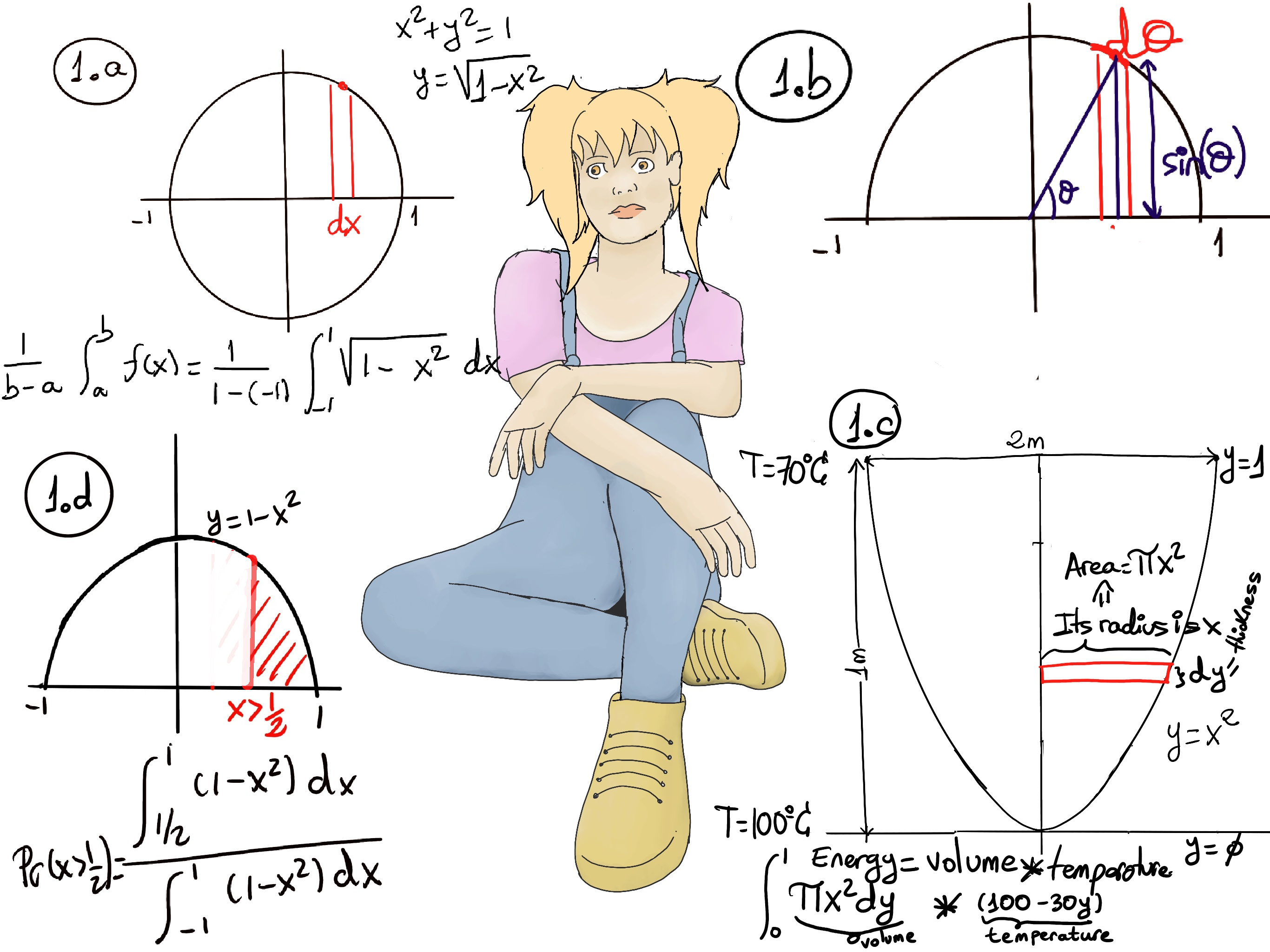
🚀 $\int_{-1}^{1} \sqrt{1-x^2}dx =$[x = sin(θ), dx = cos(θ)dθ, x = -1 ⇒ θ = -π/2; x = 1, θ = π/2] $\int_{-\frac{π}{2}}^{\frac{π}{2}} \sqrt{1-sin^2(θ)}cos(θ)dθ = \int_{-\frac{π}{2}}^{\frac{π}{2}} cos^2(θ)dθ$ = [cos(2θ) = 2cos²(θ) -1] $\frac{1}{2}\int_{-\frac{π}{2}}^{\frac{π}{2}} (1 +cos(2θ))dθ = \frac{1}{2}(θ + \frac{1}{2}sin(2θ))\bigg|_{-\frac{π}{2}}^{\frac{π}{2}} = \frac{1}{2}(\frac{π}{2} + \frac{1}{2}sin(π)- (-\frac{π}{2} + \frac{1}{2}sin(-π))) = \frac{π}{2}$.

• Find the average function value for f(x) = 16 -x² over [-4, 4] and determine the values for which the function equals its average value.

f_avg = $\frac{\int_{a}^{b} f(x)dx}{b-a} = \frac{\int_{-4}^{4} (16-x^2)dx}{4-(-4)}=$ [Integral of an even function] $\frac{2}{8}\int_{0}^{4} (16-x^2)dx = \frac{1}{4}·(16x -\frac{x^3}{3}\bigg|_{0}^{4}) = \frac{1}{4}·(16·4-\frac{4^3}{3}) = \frac{1}{4}·(64-\frac{64}{3}) =\frac{1}{4}·(64\frac{3}{3}-\frac{64}{3}) = \frac{1}{4}·64·\frac{2}{3} = \frac{32}{3}$

To determine the values for which the function equals its average value, $\frac{32}{3}$, $16-x^2 =\frac{32}{3} ↭ -x^2 = \frac{32}{3} -\frac{48}{3} ↭ -x^2 = \frac{-16}{3} ↭ x^2 = \frac{16}{3} ↭ x = ±\sqrt{\frac{16}{3}} = ±\frac{4}{sqrt(3)} ≈ 2.3094$.

• Find the average height of a point on a unit semicircle with respect to the arc length θ.

The arc length θ can be related to the angle formed by the point on the semicircle and the positive x-axis. Let’s denote this angle as α. When the radius is 1 (The arc length is the angle times the radius), the arc-length is the angle.

$\frac{1}{b-a}\int_{a}^{b} f(x)dx = \frac{1}{π}\int_{0}^{π} sin(θ)dθ = -\frac{1}{π}cos(θ)\bigg|_{0}^{π} = -\frac{1}{π}(cos(π)-cos(0)) = -\frac{1}{π}(-2) = \frac{2}{π}$ (Figure 1.b).

• Find the average function value for f(x) = (x-5)² over [4, 7] and determine the values for which the function equals its average value.

f_avg = $\frac{\int_{a}^{b} f(x)dx}{b-a} = \frac{\int_{4}^{7} (x-5)^2dx}{7-4} = \frac{\int_{4}^{7} (x^2-10x+25)dx}{3} = \frac{1}{3}(\frac{x^3}{3}-10\frac{x^2}{2}+25x)dx = \frac{1}{3}(\frac{x^3}{3}-5x^2+25x)dx\bigg|_{4}^{7} = \frac{1}{3}(\frac{7^3}{3}-5·7^2+25·7-(\frac{4^3}{3}-5·4^2+25·4)) = \frac{1}{3}(\frac{133}{3}-\frac{124}{3}) = \frac{1}{3}·\frac{9}{3} = 1$

To determine the values for which the function equals its average value, 1, $(c-5)^2 = 1 ↭ \sqrt{(c-5)^2} = ±\sqrt{1}↭ c - 5 = ± 1 ↭$ c = 4 or c = 6 and both values of c are in the given closed interval.

Bibliography

1. NPTEL-NOC IITM, Introduction to Galois Theory.
2. Algebra, Second Edition, by Michael Artin.
3. LibreTexts, Calculus. Abstract and Geometric Algebra, Abstract Algebra: Theory and Applications (Judson).
4. Field and Galois Theory, by Patrick Morandi. Springer.
5. Michael Penn, and MathMajor.
6. Contemporary Abstract Algebra, Joseph, A. Gallian.
7. YouTube’s Andrew Misseldine: Calculus. College Algebra and Abstract Algebra.
8. MIT OpenCourseWare 18.01 Single Variable Calculus, Fall 2007 and 18.02 Multivariable Calculus, Fall 2007.
9. Calculus Early Transcendentals: Differential & Multi-Variable Calculus for Social Sciences.