Absolute Extrema

“Beauty is so many things… and you are in all of them,” I said. “Wow! I thought that you were as romantic as a brick to the head or a nuclear bomb’s guts”, Susanne replied, Apocalypse, Anawim, #justothepoint.

“When bias narratives, misleading news without context, and blatant lies spread like wildfire, history needs to be rewritten and truth needs to be cancelled”, Anawim, #justothepoint.

Recall

Definition. A function f is a rule, relationship, or correspondence that assigns to each element of one set (x ∈ D), called the domain, exactly one element of a second set, called the range (y ∈ E).

The pair (x, y) is denoted as y = f(x). Typically, the sets D and E will be both the set of real numbers, ℝ.

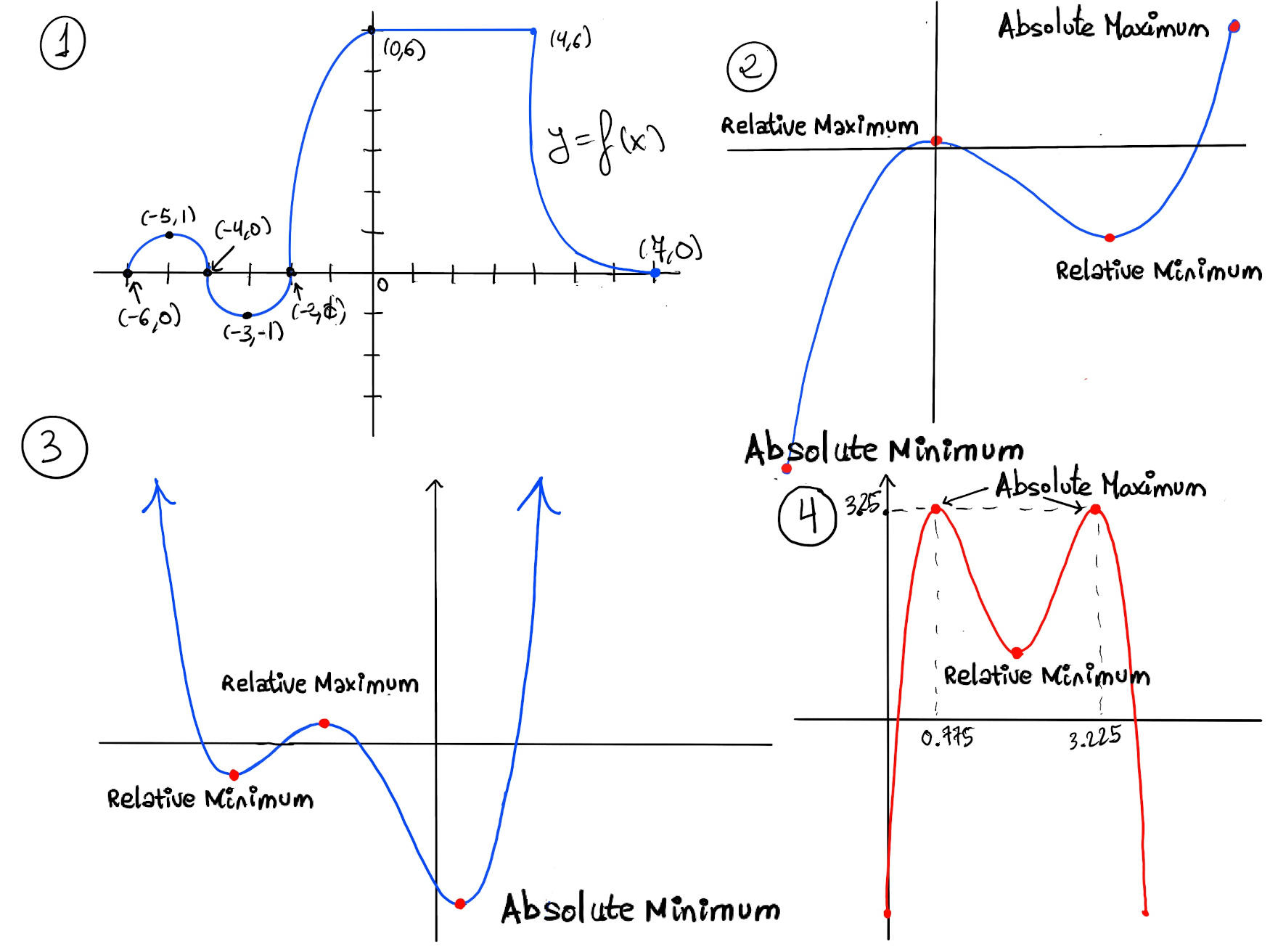
Local extrema are the largest or smallest values locally, that is, on an interval around the point. More formally, they are critical points on the graph of a function where the function reaches a maximum or minimum value (a valley) within a specific neighborhood of that point.

A critical point of a function f(x) occurs where the derivative f’(x) is either zero or undefined.

Definition. Let D be the domain of a function and c ∈ D. f is said to have a local or relative maximum point at c if there exists an interval (a, b) containing c such that f(c) is the maximum value of f on (a, b) ∩ D: f(c) ≥ f(x) ∀ x in (a, b) ∩ D.

Conversely, f is said to have a local or relative minimum point at c if there exists an interval (a, b) containing c such that f(c) is the minimum value of f on (a, b) ∩ D: f(c) ≤ f(x) ∀ x in (a, b) ∩ D.

Absolute Extrema

The maxima and minima (the respective plurals of maximum and minimum) of a function are the largest and smallest values of the function, either within a given range (the local or relative maximum and minimum; you can visualize them as kind of local hills and valleys), or on the entire domain (the global or absolute maximum and minimum). These points represent the global extremes of the function.

Formally, a function f defined on a domain D has a global or absolute maximum point at c if f(c) ≥ f(x) ∀ x ∈ D. Similarly, the function f has a global or absolute minimum point at c if f(c) ≤ f(x) ∀ x ∈ D.

Figure 3 illustrates a function that does not have an absolute maximum. Besides, a graph can only have one absolute minimum or maximum, but multiples x-values could obtain them as it is illustrated on figure 4. The function has an absolute maximum value of y = 3.25 which is obtained both at x = 0.775 and 3.225.

Finding absolute extrema

To find absolute extrema or the extrema of a continuous function f on a closed interval [a, b], one needs to consider critical points and endpoints of the domain, and use the following steps.

1. Verify that the function is continuous.
2. Compute its critical points. A critical point occurs where the derivative is either zero or undefined (set f’(x) = 0 and solve for x).
3. Evaluate f at each critical number.
4. Check the values of f’(x) around these critical points to determine whether it changes sign, indicating a possible extremum.
5. Evaluate the function at the endpoints of the domain or [a, b].
6. Take into consideration the Extreme Value Theorem. It states that if a real-valued function f is continuous on the closed interval [a,b], then f must have at least one maximum value and one minimum value on that interval.
7. Identify the absolute extrema.

Solved examples

• Find the extrema of f(x) = 3x⁴ -4x³ on the interval [-1, 2] (figure 2.a).

f’(x) = 12x³ -12x². f’(x) = 0 ↭ 12x³ -12x² = 0 ↭ x²(12x -12) ⇒ x = 0, 1 are the critical points. Evaluate the function at these critical points: (0, 0) and (1, -1).

Evaluate the function at the endpoints of [-1, 2]: (-1, 7), (2, 16).

Conclusion. The local or relative maximum on [-1, 2]: (2, 16). The local or relative minimum on [-1, 2]: (1, -1).

• Find the extrema of f(x) = ln(x)·x² (Figure 2.b).

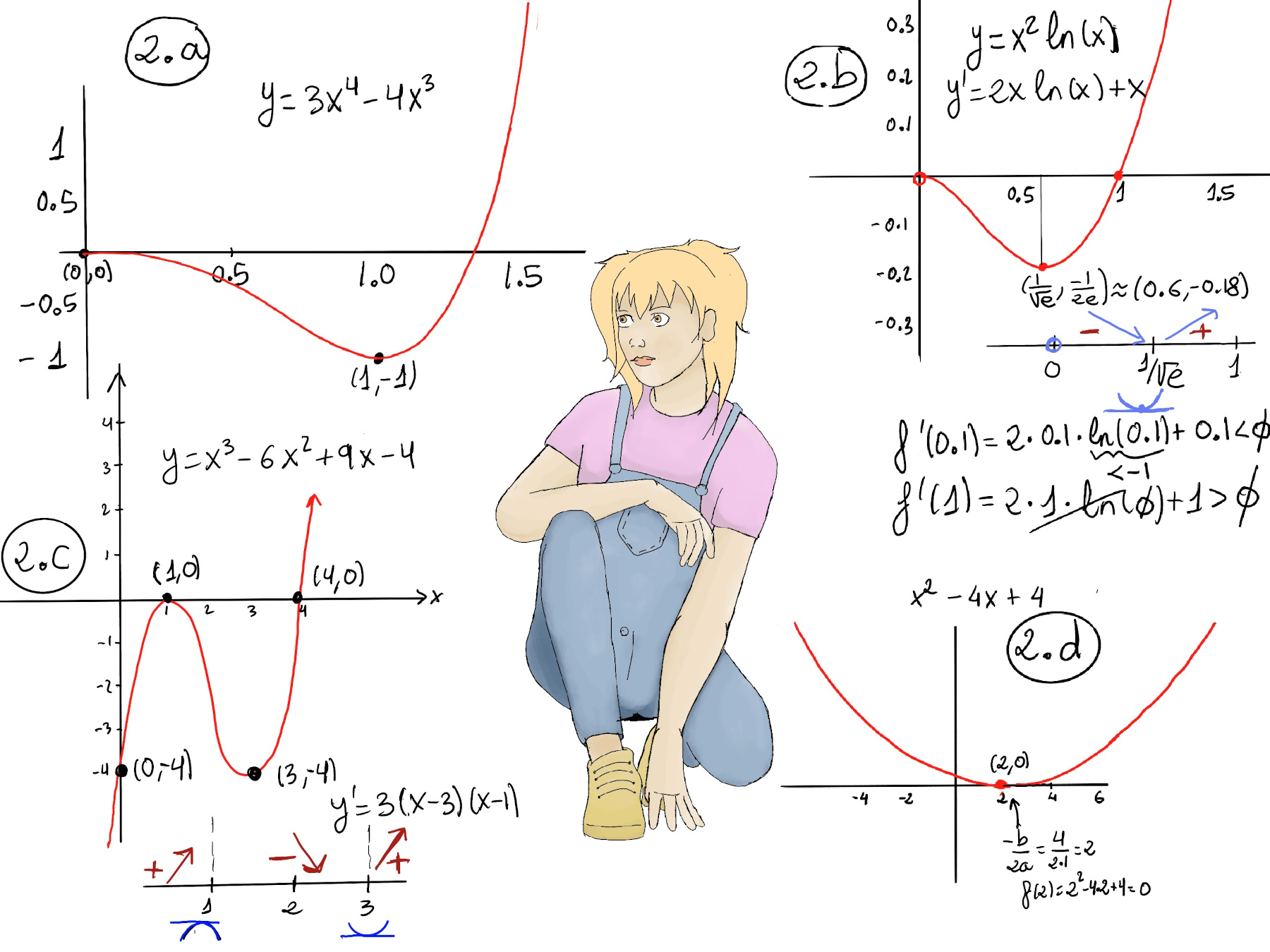
Domain: x > 0. f’(x) = 2xln(x) + x.

f’(x) = 0 ↭ 2xln(x) + x = 0 ↭[x > 0] 2ln(x) + 1 = 0 ↭ ln(x) = ^-1⁄₂, x = $e^{\frac{-1}{2}} = \frac{1}{\sqrt{e}}$ ≈ 0.6. Besides, $f(e^{\frac{-1}{2}}) = \frac{-1}{2}·e^{-1} = \frac{-1}{2e}$, so the point is approximately (0.6, -0.18).

Conclusion. There’s no global or absolute maximum point, but a global or absolute minimum point at $(\frac{1}{\sqrt{e}}, \frac{-1}{2e})$.

• Find the extrema of f(x) = x³ -6x² +9x -4 (Figure 2.c).

The avid reader could notice that x³ -6x² +9x -4 = (x-4)(x-1)².

Domain: (-∞, +∞). f’(x) = 3x² -12x +9.

f’(x) = 0 ↭ 3x² -12x +9 = 0 ↭ 3(x²) -4x + 3 = 0 ↭ 3(x -3)(x -1) = 0 ⇒ x = 3, 1 are the critical points. Evaluate the function at these critical points: (1, 0), (3, -4).

However, $\lim_{x \to ∞} x^3 -6x^2 +9x -4= ∞, \lim_{x \to ∞} x^3 -6x^2 +9x -4= -∞$ ⇒ (1, 0) is a local maximum and (3, -4) is a local minimum, but there’s no global extreme points.

Additional information. x-intercept: (4, 0) and (1, 0). y-intercept (0, -4) and we have no asymptotes.

• A parabola is a U-shaped curve that represents a quadratic function. When the parabola opens up (a > 0), the vertex symbolizes the lowest point on the graph or the quadratic function’s minimum value ($\frac{-b}{2a}, f(\frac{-b}{2a})$), e.g., x² -4x +4 has an absolute minimum at (2, 0) because a = 1 > 0 (Figure 2.d.).

Similarly, when the parabola opens down (a < 0), the vertex symbolizes the highest point on the graph or the quadratic function’s maximum value, e.g., -x²+x-6 has an absolute maximum at $(\frac{-b}{2a}, f(\frac{-b}{2a})) = (0.5, -5.75)$ because a = -1 < 0.

Bibliography

1. NPTEL-NOC IITM, Introduction to Galois Theory.
2. Algebra, Second Edition, by Michael Artin.
3. LibreTexts, Calculus. Abstract and Geometric Algebra, Abstract Algebra: Theory and Applications (Judson).
4. Field and Galois Theory, by Patrick Morandi. Springer.
5. Michael Penn, and MathMajor.
6. Contemporary Abstract Algebra, Joseph, A. Gallian.
7. YouTube’s Andrew Misseldine: Calculus. College Algebra and Abstract Algebra.
8. MIT OpenCourseWare 18.01 Single Variable Calculus, Fall 2007 and 18.02 Multivariable Calculus, Fall 2007.
9. Calculus Early Transcendentals: Differential & Multi-Variable Calculus for Social Sciences.